|
An
Improvement on the Gamma Graphs |
| |
|
573. |
In working with Existential Graphs, we use, or at any
rate imagine
that we use, a sheet of paper of different tints on its
two sides. Let us say
that the side we call the recto is cream white while the
verso is usually of
somewhat bluish grey, but may be of yellow or of a rose
tint or of green. The
recto is appropriated to the representation of
existential, or actual, facts,
or what we choose to make believe are such. The verso is
appropriated to the
representation of possibilities of different kinds
according to its tint, but
usually to that of subjective possibilities, or
subjectively possible truths.
The special kind of possibility here called subjective
is that which consists
in ignorance. If we do not know that there are not
inhabitants of Mars, it is
subjectively possible that there are such beings. . . .
|
| |
|
|
|
574. |
The verso is usually appropriated to imparting information about
subjective possibilities or what may be true for aught we know. To scribe a
graph is to impart an item of information; and this item of information does
one of two things. It either adds to what we know to exist or it cuts off
something from our list of subjective possibilities. Hence, it must be that a
graph scribed on the verso is thereby denied. |
| |
|
|
|
575. |
Now the denial of a subjective possibility usually, if not always,
involves the assertion of a truth of existence; and consequently what is put
upon the verso must usually have a definite connection with a place on the
recto.
|
| |
|
|
|
576. |
In my former exposition of Existential Graphs, I said that there
must be a department of the System which I called the Gamma part into which I
was as yet able to gain mere glimpses, sufficient only to show me its reality,
and to rouse my intense curiosity, without giving me any real insight into it.
The conception of the System which I have just set forth is a very recent
discovery. I have not had time as yet to trace out all its consequences. But it
is already plain that, in at least three places, it lifts the veil from the
Gamma part of the system. |
| |
|
|
|
577. |
The new discovery which sheds such a light is simply that, as the
main part of the sheet represents existence or actuality, so the area within a
cut, that is, the verso of the sheet, represents a kind of possibility. |
| |
|
|
|
578. |
From thence I immediately infer several things that I
did not understand before, as follows: First, the cut may be imagined to extend down to one or another depth
into the paper, so that the overturning of the piece cut out may expose one
stratum or another, these being distinguished by their tints; the different
tints representing different kinds of possibility.
This improvement gives substantially, as far as I can see, nearly the
whole of that Gamma part which I have been endeavoring to discern. |
| |
|
|
|
579. |
Second, In a certain partly printed but unpublished "Syllabus of
Logic," which contains the only formal or full description of Existential
Graphs that I have ever undertaken to give, I laid it down, as a rule, that no
graph could be partly in one area and partly in another;(*1) and this I said
simply because I could attach no interpretation to a graph which should cross a
cut. As soon, however, as I discovered that the verso of the sheet represents a
universe of possibility, I saw clearly that such a graph was not only
interpretable, but that it fills the great lacuna in all my previous
developments of the logic of relatives. For although I have always recognized
that a possibility may be real, that it is sheer insanity to deny the reality
of the possibility of my raising my arm, even if, when the time comes, I do not
raise it; and although, in all my attempts to classify relations, I have
invariably recognized, as one great class of relations, the class of
references, as I have called them, where one correlate is an existent, and
another is a mere possibility; yet whenever I have undertaken to develop the
logic of relations, I have always left these references out of account,
notwithstanding their manifest importance, simply because the algebras or other
forms of diagrammatization which I employed did not seem to afford me any means
of representing them.(*2) I need hardly say that the moment I discovered in the
verso of the sheet of Existential Graphs a representation of a universe of
possibility, I perceived that a reference would be represented by a graph which
should cross a cut, thus subduing a vast field of thought to the governance and
control of exact logic. |
| |
|
|
|
580. |
Third, My previous account of Existential Graphs
was marred by a certain rule which, from the point of
view from which I thought the system ought to be
regarded, seemed quite out of place and inacceptable,
and yet which I found myself unable to dispute.(*1)
I will just illustrate this matter by an example.
Suppose we wish to assert that there is a man every
dollar of whose indebtedness will be paid by some man or
other, perhaps one dollar being paid by one man and
another by another man, or perhaps all paid by the same
man. We do not wish to say how that will be. Here will
be our graph, Fig. 219. |
|
|
|
|
|
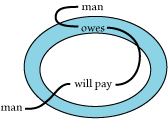
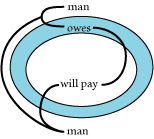
But if we wish to assert that one man will pay the
whole, without saying in what relation the payer stands
to the debtor, here will be our graph, Fig. 220. Now
suppose we wish to add that this man who will pay all
those debts is the very same man who owes them. Then we
insert two graphs of teridentity and a line of identity
as in Fig. 221. |
|
|
 |
 |
|
Figure 220 |
Figure 221 |
|
|
|
The difference
between the graph with and without this added line is obvious, and is perfectly
represented in all my systems. But here it will be observed that the graph
"owes" and the graph "pays" are not only united on the left by a line outside
the smallest area that contains them both, but likewise on the right, by a line
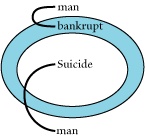
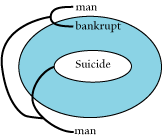
inside that smallest common area. Now let us consider a case in which this
inner connection is lacking. Let us assert that there is a man A and a man B,
who may or may not be the same man, and if A becomes bankrupt then B will
suicide. Then, if we add that A and B are the same man, by drawing a line
outside the smallest common area of the |
|
|
|
|
 |
 |
|
Figure 222 |
Figure 223 |
|
|
|
graphs joined, which are here bankrupt and suicide, the strange rule to which I
refer is that such outer line, because there is no connecting line within the
smallest common area, is null and void, that is, it does not affect the
interpretation in the least. . . . The proposition that there is a man who if
he goes bankrupt will commit suicide is false only in case, taking any man you
please, he will go bankrupt, and will not suicide. That is, it is falsified
only if every man goes bankrupt without suiciding. But this is the same as the
state of things under which the other proposition is false; namely, that every
man goes broke while no man suicides. This reasoning is irrefragable as long as
a mere possibility is treated as an absolute nullity. Some years ago,(*3)
however, when in consequence of an invitation to deliver a course of lectures
in Harvard University upon Pragmatism, I was led to revise that doctrine, in
which I had already found difficulties, I soon discovered, upon a critical
analysis, that it was absolutely necessary to insist upon and bring to the
front, the truth that a mere possibility may be quite real. That admitted, it
can no longer be granted that every conditional proposition whose antecedent
does not happen to be realized is true, and the whole reasoning just given
breaks down. |
| |
|
|
|
581. |
I often think that we logicians are the most obtuse of men, and
the most devoid of common sense. As soon as I saw that this strange rule, so
foreign to the general idea of the System of Existential Graphs, could by no
means be deduced from the other rules nor from the general idea of the system,
but has to be accepted, if at all, as an arbitrary first principle -- I ought
to have asked myself, and should have asked myself if I had not been afflicted
with the logician's bÍtise, What compels the adoption of this rule? The answer
to that must have been that the interpretation requires it; and the inference
of common sense from that answer would have been that the interpretation was
too narrow. Yet I did not think of that until my operose method like that of a
hydrographic surveyor sounding out a harbour, suddenly brought me up to the
important truth that the verso of the sheet of Existential Graphs represents a
universe of possibilities. This, taken in connection with other premisses, led
me back to the same conclusion to which my studies of Pragmatism had already
brought me, the reality of some possibilities. This is a striking proof of the
superiority of the System of Existential Graphs to either of my algebras of
logic.(*1)
For in both of them the incongruity of this strange rule is completely
hidden behind the superfluous machinery which is introduced in order to give an
appearance of symmetry to logical law, and in order to facilitate the working
of these algebras considered as reasoning machines. I cannot let this remark
pass without protesting, however, that in the construction of no algebra was
the idea of making a calculus which would turn out conclusions by a regular
routine other than a very secondary purpose. . . .(*2) |
| |
|
|
|
582. |
The sheet of the graphs in all its states collectively, together
with the laws of its transformations, corresponds to and represents the Mind in
its relation to its thoughts, considered as signs. That thoughts are signs has
been more especially urged by nominalistic logicians; but the realists are, for
the most part, content to let the proposition stand unchallenged, even when
they have not decidedly affirmed its truth. The scribed graphs are
determinations of the sheet, just as thoughts are determinations of the mind;
and the mind itself is a comprehensive thought just as the sheet considered in
all its actual transformation-states and transformations, taken collectively,
is a graph-instance and taken in all its permissible transformations is a
graph. Thus the system of existential graphs is a rough and generalized diagram
of the Mind, and it gives a better idea of what the mind is, from the point of
view of logic, than could be conveyed by any abstract account of it.
|
| |
|
|
|
583. |
The System of Existential Graphs recognizes but one mode of
combination of ideas, that by which two indefinite propositions define, or
rather partially define, each other on the recto and by which two general
propositions mutually limit each other upon the verso; or, in a unitary
formula, by which two indeterminate propositions mutually determine each other
in a measure. I say in a measure, for it is impossible that any sign whether
mental or external should be perfectly determinate. If it were possible such
sign must remain absolutely unconnected with any other. It would quite
obviously be such a sign of its entire universe, as Leibniz and others have
described the omniscience of God to be, an intuitive representation amounting
to an indecomposable feeling of the whole in all its details, from which those
details would not be separable. For no reasoning, and consequently no
abstraction, could connect itself with such a sign. This consideration, which
is obviously correct, is a strong argument to show that what the system of
existential graphs represents to be true of propositions and which must be true
of them, since every proposition can be analytically expressed in existential
graphs, equally holds good of concepts that are not propositional; and this
argument is supported by the evident truth that no sign of a thing or kind of
thing -- the ideas of signs to which concepts belong -- can arise except in a
proposition; and no logical operation upon a proposition can result in anything
but a proposition; so that non-propositional signs can only exist as
constituents of propositions. But it is not true, as ordinarily represented,
that a proposition can be built up of non-propositional signs. The truth is
that concepts are nothing but indefinite problematic judgments. The concept of
man necessarily involves the thought of the possible being of a man; and thus
it is precisely the judgment, "There may be a man." Since no perfectly
determinate proposition is possible, there is one more reform that needs to be
made in the system of existential graphs. Namely, the line of identity must be
totally abolished, or rather must be understood quite differently. We must
hereafter understand it to be potentially the graph of teridentity by which
means there always will virtually be at least one loose end in every graph. In
fact, it will not be truly a graph of teridentity but a graph of indefinitely
multiple identity. |
| |
|
|
|
584. |
We here reach a point at which novel considerations about the
constitution of knowledge and therefore of the constitution of nature burst in
upon the mind with cataclysmal multitude and resistlessness. It is that
synthesis of tychism and of pragmatism for which I long ago proposed the name,
Synechism,(*1) to which one thus returns; but this time with stronger reasons
than ever before. But I cannot, consistently with my own convictions, ask the
Academy to listen to a discourse upon Metaphysics. |
| |
|
|
|
|
| |